Vol.:(0123456789)

1 3

Acta Metallurgica Sinica (English Letters)

https://doi.org/10.1007/s40195-020-01090-4

Evolution ofAnnealing Twins andRecrystallization Texture

inThin‑Walled Copper Tube During Heat Treatment

Song‑WeiWang

1,2

· Hong‑WuSong

· YanChen

· Shi‑HongZhang

1,2

· Hai‑HongLi

Received: 5 January 2020 / Revised: 25 February 2020 / Accepted: 1 April 2020

Abstract

Thin-walled copper tubes are usually produced by multi-pass ﬂoat-plug drawing deformation. In general, the annealing

treatment subsequently is necessary to release the stored energy and adjusts the microstructure. In this study, an investiga-

tion on the evolution of annealing twins as well as textures in the thin-walled (Ф6mm × 0.3mm) copper tube underwent

holding time-free heat treatment was reported. Electron backscattered diﬀraction analysis reveals that a large number of

Σ3 boundaries (60° 〈111〉 twin relationship) are produced at the early stage of heat treatment, which is due to the lower

boundary energy. With the recrystallization proceeding, the migration rate of grain boundaries decreases on account of the

grain growth; meanwhile, the unique Σ9 boundaries (38.9° 〈110〉 relationship) are formed due to the interaction of the Σ3

boundaries. As a result, the number fractions of Σ3 boundaries and high-angle grain boundaries decrease rapidly. During

the grain growth stage, a strong recrystallization texture was formed due to the fact that the grains of Goss orientation have a

growth advantage over the others. As a result, the initial copper texture was transferred into the Goss texture in domination.

Keywords Copper tube· Annealing twin· Heat treatment· Electron backscattered diﬀraction (EBSD) analysis·

Recrystallization texture

1 Introduction

Since Carpenter and Tamura [1] ﬁrstly observed the forma-

tion of annealing twins in 1926, it has been found massively

in the deformed and then annealed materials. Especially in

the face-center cubic (FCC) metals with low or medium

stacking fault energies (SFE) (i.e., Cu, Ag, and Ni), anneal-

ing twins are frequently observed [2–4]. According to Bran-

don’s criterion, the twin boundary in FCC crystal structure

is characterized by rotating the orientation of the parent

grain 60° about 〈111〉 axis [5]. The twin boundaries are also

deﬁned as ∑3 boundaries that lies in the (111) plane of both

crystals, which is one of the special boundaries exploited in

grain boundary engineering [6–8]. The annealing twins in

these metals with a variety of orientations will signiﬁcantly

aﬀect the microstructures and textures, as well as the prop-

erties, i.e., strengthening of the nanostructured Cu [9], the

nucleation of fatigue cracks [10], and the stagnation of grain

growth [11].

The forming mechanism of annealing twins during

recrystallization and the subsequently evolution during

grain growth process are still under research. There are

three main mechanisms for twin formation proposed by

some researchers. The ﬁrst theory thought that the crystals

already have a twin relationship impinge during growth and

then formed the twins [12, 13]. The second one thought

that the twin forms when a misplaced layer of atoms on the

(111) plane due to the low stacking fault energy [14–17].

The last theory assumed that the grain boundaries with

higher energy transform into a twin boundary and lower

energy grain boundaries [18–21]. Therefore, the evolution

of annealing twin continuously takes place during recrys-

tallization, and the available evidence cannot discriminate

these theories. The availability of recent techniques such as

Available online at http://link.sprin ger.com/journ al/40195 .

* Shi-Hong Zhang

shzhang@imr.ac.cn

Institute ofMetal Research, Chinese Academy ofSciences,

Shenyang110016, China

School ofMaterials Science andEngineering, University

ofScience andTechnology ofChina, Shenyang100049,

China

School ofNuclear Equipment andNuclear Engineering,

Yantai University, Yantai264005, China

S.-W.Wang et al.

1 3

electron backscattered diﬀraction (EBSD) makes it possible

to investigate and understand the potential sources for the

behaviors found in earlier works. Nowadays, the microstruc-

tural behavior and annealing twin evolution in nickel [4,

22], nickel-based superalloy [23, 24], and super-austenitic

steel [25] were investigated by EBSD method. The former

researches also indicated that not only the materials but also

the predeformation process and initial deformation texture

are essential for the microstructural evolution during anneal-

ing process.

With an aim to understand the recrystallization behavior

during the heat treatment, the studies have been carried out

to ﬁgure out the evolution of texture and the microstructure

in pure copper. The eﬀect of cold rolling process on texture

evolution of pure copper has been well investigated [26–29]

that shows the cube orientation as a recrystallization texture

and the formation mechanisms have been proposed as the

oriented growth theory. Anand etal. [30] characterized the

annealed pure copper sheet after cryo-rolling process and

found that with the increase of annealing time, the sample

shows a weakening of Brass texture, then exhibits almost

random texture, and ﬁnally forms the strong cube texture,

while the cold-rolled Al-0.3% Cu develops a strong Goss

texture during annealing treatment which is related to the

oriented nucleate mechanism [31]. Choi etal. [32] proposed

the strain energy release maximization model to predict the

texture changes of copper electrodeposits after recrystalliza-

tion. Also, the cold-drawn copper wires have been studied

by Baudin etal. [33] after recrystallization, and the results

showed the 〈100〉 ﬁber increases at the expense of the other

texture components. Nowfal etal. [34] used the synchrotron

diﬀraction method to investigate the local texture gradient

through the wall thickness of SF-Cu tubes with an average

wall thickness of 10mm. The structure consists of recrystal-

lized equiaxed grains with twins, and the dominant texture

component is the {001} 〈100〉 cube component.

To the knowledge of the authors, little eﬀorts were taken

to study the annealing twins and recrystallization textures

of the thin-walled copper tubes. The precision copper tube

with a diameter of 6mm and a wall thickness of 0.3mm

was manufactured by the ﬂoat-plug drawing process, which

exhibited a unique deformation microstructure and textures

[35, 36]. The copper tube was widely used in refrigeration

industries, due to its excellent thermal conductivity and plas-

ticity. Usually, a heat treatment process is necessary after

the deformation in order to release the stored energy of cold

work. Thus, the microstructural evolution during recrystal-

lization process is crucial for the ﬁnal properties [37, 38],

such as fatigue resistance, corrosion resistance, and mechan-

ical properties. The purpose of this study is to investigate

the annealing twin boundaries as well as recrystallization

texture of the copper tube during the heat treatment. Firstly,

an evolving structure was obtained by taking the samples at

diﬀerent heating stages. Then, the EBSD technology was

utilized to analyze the evolution of microstructure and the

textures during the recrystallization process.

2 Experimental

Highly puriﬁed oxygen-free copper tube billet (99.997%

pure) was deformed by ﬂoat-plug drawing process to obtain

a Ф6 × 0.3mm ﬁnal tube as an initial material. The initial

tube was cut into the segments about 200mm long, and

the segments were vacuum packaged with quartz tube to

prevent oxidation of surface. Then, the heat treatment was

carried out on the segments by using the electric resistance

furnace, from room temperature to 900 °C without holding

time. To examine the evolution of microstructure, the single

segment was taken out at 200 °C, 300 °C, 400 °C, 500 °C,

700 °C, and 900 °C, respectively, and then water-quenched

immediately to maintain the microstructure.

The annealing microstructures were analyzed by EBSD.

The samples with size of 5mm in length (drawing direction,

DD), 3mm in width (circumferential direction, CD), and

0.3mm in thickness (radial direction, RD) were cut from the

wall thickness of annealed tubes, seen as Fig.1. The local

coordinate system used to identify the direction of inverse

pole ﬁgure (IPF) and pole ﬁgure (PF) is also presented in

Fig.1. The x-, y-, and z-axis correspond to DD, CD, and RD

direction, respectively. The RD direction is the normal direc-

tion of the observation surface (DD-CD). The DD-CD sec-

tion was ground slightly to obtain a smooth plane and then

polished with diamond paste and ﬁnished with ion milling

to remove the surface deformation layer. EBSD examina-

tions were carried out using a FEI Nova Nano SEM 430

ﬁeld-emission scanning electron microscope equipped with

a fully automatic HKL Technology EBSD attachment oper-

ated at 20kV. The HKL Channel 5 software was used for the

EBSD information collection and characterization. EBSD

maps were acquired using step sizes of 0.12–0.5µm to ana-

lyze the twins and substructures in the observation plane

Fig. 1 Observation surface of the samples for EBSD measurement

Evolution ofAnnealing Twins andRecrystallization Texture inThin-Walled Copper Tube During…

1 3

containing the drawing direction and circumferential direc-

tion (DD-CD). In this study, a critical misorientation angle

of 2° was applied to observe boundaries in the orientation

maps, where low-angle grain boundaries (LAGBs) and high-

angle grain boundaries (HAGBs) were deﬁned as boundaries

between grains with misorientation 2–10° and > 10°, respec-

tively. The average grain size was obtained by using Atex

software with twins not included as separate grains. And

the Atex software was also used to determine the volume

fractions of diﬀerent texture components, within a spread

of 15° around their respective ideal locations in Euler space.

3 Results andAnalysis

3.1 Microstructure Evolution

Figure2 reveals the initial microstructure of drawn tube

characterized by the orientation map, and it is visible that

the grains were elongated and ﬁbered after the drawing

deformation. Figure2a shows the distribution of grain size

on the observation surface, with a minimum of 1.28μm

and a maximum of 45.05μm. Figure2b shows deforma-

tion texture in the initial tube, a large proportion of copper

{112} 〈111〉 component, and a weak Goss {110} 〈001〉

component. Previous studies [36] have reported the texture

evolution of copper tube and its relationship with the area

reduction during ﬂoating plug drawing process.

The EBSD maps character from the copper tube sam-

ples after heat treatment at temperatures ranging from 200

°C to 900 °C (without holding time due to the thin wall

thickness) is presented in Fig.3. Figure3a, b shows that

the microstructure remains the drawn ﬁber at 200 and 300

°C, and only a few recrystallization nucleuses are observed

in the stretched grains. Figure3c, d shows a large number

of ∑3 boundaries generated at 400–500 °C, and it can

be seen that the recrystallization grains grow up signiﬁ-

cantly. When the temperature reaches to 700 °C, as shown

in Fig.3e, recrystallized grains occupy the initial micro-

structure. The annealing twins grow with the growth of the

recrystallized grains. When the temperature increases to

900 °C, as seen in Fig.3f, the grains seriously coarsen that

leads to the broaden of the annealing twins in it.

Fig. 2 a EBSD orientation maps of the drawn tube, b texture components on (111) pole ﬁgure

S.-W.Wang et al.

1 3

In order to investigate the evolution of recrystallization

grains and annealing twins during heat treatment, further

analysis was carried on the microstructural parameters,

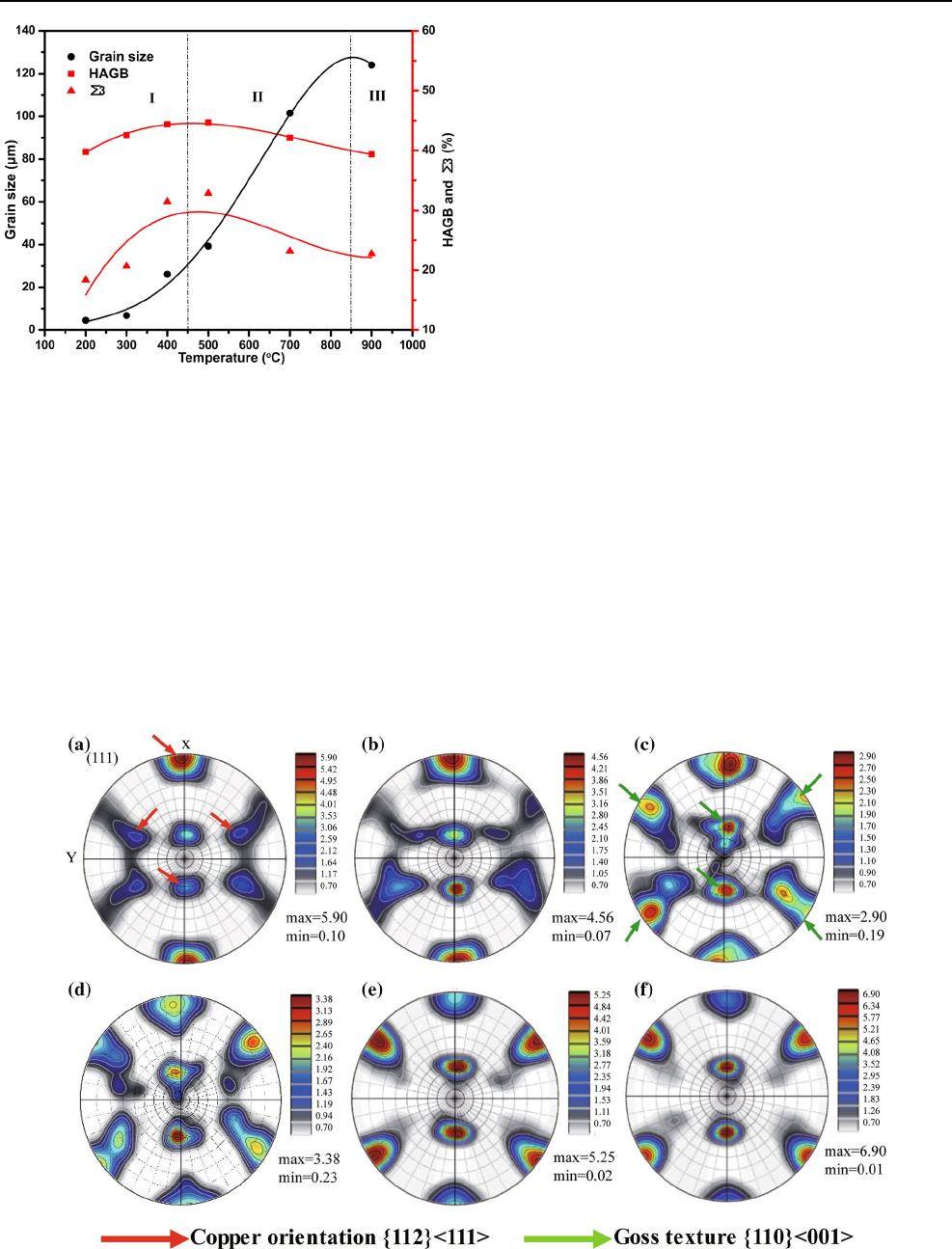
as shown in Fig.4. According to the shape of curves, the

results were divided into three stages using the vertical

dashed lines. This can roughly represent the (I) nucleation,

(II) normal grain growth, and (III) abnormal grain growth.

During stage I, the fraction of high-angle grain boundary

and ∑3 boundaries increases sharply, and the average grain

size (without twins) increases slightly ﬁrst and then rises

quickly. In stage II, it is seen that the grain size continues

to rise at a greater rate, while the HAGBs and ∑3 bounda-

ries decrease slowly. When the temperature comes near 900

°C, the grain size increases slowly and the HAGBs and ∑3

boundaries tend to be stable. It is obvious that the evolution

of HAGBs content with temperature has the same tendency

with ∑3 boundaries.

3.2 Texture Evolution

In order to examine the orientation of recrystallization

grains during heat treatment, pole ﬁgure (PF) was calcu-

lated by using Atex software. Figure5 shows the creation

and evolution of diﬀerent texture components. As shown

in Fig.5a, two texture components can be observed in

the initial drawn tube, which are marked as Goss compo-

nent {110} 〈001〉 and copper component {112} 〈111〉.

And the intensity of copper component is much higher

Fig. 3 EBSD maps from the observation plane (DD-CD) of the tubes annealed at: a 200 °C, b 300 °C, c 400 °C, d 500 °C, e 700 °C, f 900 °C.

Black lines and red lines show HAGBs and Σ3 boundaries, respectively

Evolution ofAnnealing Twins andRecrystallization Texture inThin-Walled Copper Tube During…

1 3

than the other one. During the heat treatment process, the

maximum intensity of texture decreased ﬁrst (from 5.9 to

2.9) and then increased again (from 2.9 to 6.9), and the

turning point is at 400 °C. Along with this tendency, it is

corresponding to the weakening of copper component and

enhancing of Goss component. Thus, when the tempera-

ture is 900 °C, Goss texture is the main recrystallization

texture in the thin-walled copper tube.

4 Discussion

4.1 Formation oftheAnnealing Twins

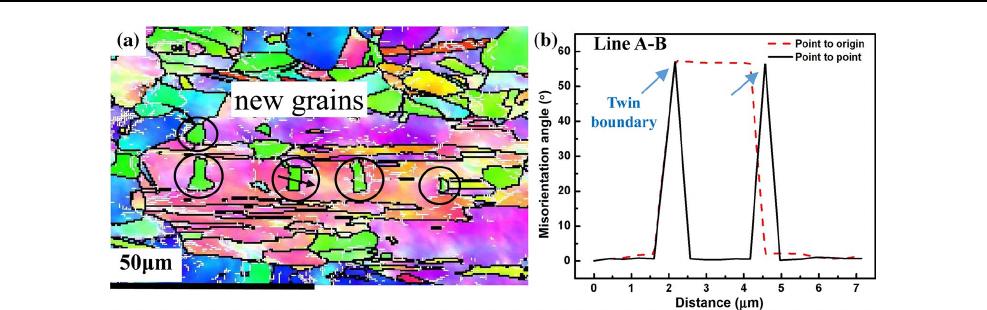
At the ﬁrst stage of heat treatment, it is clearly observed

that a large number of annealing twins are formed. In order

to examine the nucleation of annealing twins, high-mag-

niﬁcation EBSD was applied on the local position in 300

°C (Fig.3b, Position 1), as shown in Fig.6, the black thick

line represents the high-angle grain boundaries (HAGBs),

while the thin white line represents low-angle grain bounda-

ries (LAGBs). The new grains were largely formed in the

stretched grains, and it seems that the nucleation gener-

ated at the grain boundaries and grew toward to inner of

deformed grains. Figure6b shows the line proﬁle along the

black arrow across the new grain in Fig.6a, and it indicated

that the new grain has a twin-orientated relationship (60°

〈111〉) with deformed matrix. Similar orientation relation-

ship had been reported in some other works [39–41], and it

is observed that when a nucleus is formed, it may continue to

grow by twinning. It is known that the formation of anneal-

ing twins is related to the “growth accident,” the coherent

twin boundaries generate at migrating grain boundaries due

to the stacking errors [42]. Therefore, the annealing twin is

likely to form in the FCC metals with low stacking faults

energy. Also, the total boundary energy theory (i.e., the

energy per unit length of both the grain and twin boundary)

Fig. 4 Evolution of microstructural parameters for the tube samples

during heat treatment

Fig. 5 (111) pole ﬁgure of samples at various annealing conditions: a 200 °C, b 300 °C, c 400 °C, d 500 °C, e 700 °C, f 900 °C

S.-W.Wang et al.

1 3

can be used to explain why a large number of twins are

favorable to exist in the annealing copper. The reduction of

energy per unit length,

ΔE

, can be estimated using following

equation [39]:

where the

𝜎

and

𝜎

are the energy of the grain bound-

ary and the twin boundary, and the

ΔL

and

ΔL

are the

change in lengths of the grain boundary and twin bound-

ary, respectively. During the annealing treatment, there is

a reduction in boundary energy [39], and it is reported that

in Cu the twin boundary energy is only 4% of the normal

high-angle grain boundary energy [43]. As a result, the for-

mation of twin grain boundaries will minimize the energy

of grain boundaries. This may explain why it is energetically

favorable to keep many twins in copper during annealing.

However, in the drawn tube, the nucleation is accomplished

by the formation of annealing twins alongside the bulged

elongated grains, which had highly density of LAGBs,

instead of the triple junctions [4, 44]. Therefore, the frac-

tion of HAGBs and ∑3 boundaries increased sharply at the

early stage of the heat treatment.

4.2 Evolution ofTwin Boundaries During Grain

Growth Process

At the stage II of heat treatment, the grain size increased

signiﬁcantly with the increasing of temperature; meanwhile,

the fraction of HAGBs and ∑3 boundaries reached the peak

value and then decreased slowly. It is easy to understand

that the formation of annealing twin requires a moving grain

boundary [15, 16, 20], and it will reﬂect on the growth of

grain size during heat treatment. When the temperature

continues to increase, the grown grains begin to encounter

with each other, leading to a decrease of grain boundary

migration rate [45]. Therefore, it is diﬃcult for the further

(1)

ΔE =

𝜎

ΔL

𝜎

ΔL

development of annealing twins. This may be one reason for

the drop of density of ∑3 boundaries.

Another reason is the interaction eﬀect of the encoun-

tered ∑3 boundaries. It is known that the annealing twins

generated randomly alongside the deformed grain bounda-

ries, and as the temperature increased, the twins continue to

growth and encounter with the adjacent ones. Then the new

∑9 boundaries (38.9° 〈110〉 relationship) are expected to

be produced due to the interaction of two encountered ∑3

twin boundaries as depicted by the following rule [46]:

This reaction can also be found in the present heat

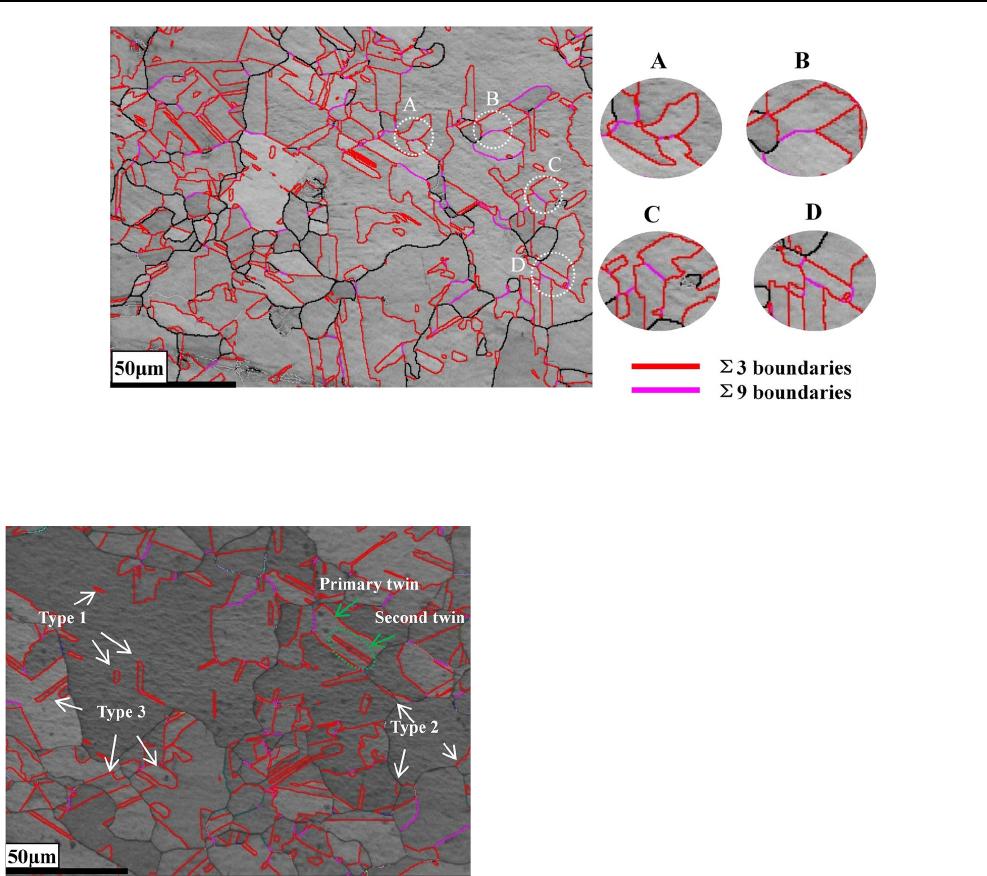
treatment, and the microstructure at 400 °C (Position 2 in

Fig.3c) was taken as an example seen in Fig.7. The HAGBs

are illustrated in black lines, the LAGBs in gray lines, the

∑3 boundaries in red lines, and the ∑9 boundaries in pink

lines. It is noticed that the boundaries of recrystallized grains

are curved and disturbed by the random ∑3 boundaries.

The LAGBs were mostly absorbed by the growth of ∑3

boundaries and normal grain boundaries. The ellipse of A,

B, C, and D illustrates the transition process. The calculated

results by the Channel 5 software showed that ∑9 boundary

fraction is only 0.184% at 300 °C and then reaches 5.24%

at 400 °C and 3.76% at 500 °C, respectively. That is to say,

these unique ∑9 misorientation relationships may ﬁnally

transform into normal HAGBs with the further migration

of the twin boundaries [47].

When the grain growth proceeds, the grain boundaries are

gradually straightened, and accordingly, the attached anneal-

ing twins continuously change with the migration of the

grain boundaries. It is obviously that there are three types

annealing twins observed at 500 °C, as shown in Fig.8, the

magniﬁcation of Position 3 in Fig.3d. The twin of the type

1 is an island left inner recrystallization grains, the type 2 is

the corner twin with a coherent boundary, and the type 3 is

(2)

∑

3 +

∑

↔

∑

Fig. 6 EBSD map of the local position 1 in Fig.3b: a the recrystallized grains, b the misorientation angle along the black arrow. The HAGBs

and LAGBs are presented by black and gray lines, respectively

Evolution ofAnnealing Twins andRecrystallization Texture inThin-Walled Copper Tube During…

1 3

the band-like twin that spans opposite sides of the grain or

terminates within the grain. The type 1 twins almost were

nonexistent when the heat-treated temperature reaches to

700 °C as seen in Fig.3e. It is assumed that the size of island

twins would decrease slowly without energy support and

ﬁnally have been fully absorbed by the growing grains. But

the type 2 twins were produced continuously at the triple

junction between grains [43], as seen in Fig.8, because of

the lower grain boundaries energy. The moving direction

of the coherent twin boundaries is toward inner grain and

lengthens with the grain growth. The primary type 3 twins

become thickening and lengthening with the growth of the

recrystallization grains and gradually become dominate at

the grain growth stage, as shown in Fig.3e, f. Also, it is

noted that new twins of type 3 occurred alongside the recrys-

tallized grain boundaries, and especially, within a primary

twin, which are marked in green circle in Fig.8. These new

twins of type 3 generated during the grain growth period

may be called second-order annealing twins, but only with

a small fraction. This can explain the stabilization of ∑3

boundaries fraction at stage III.

4.3 Eect oftheInteraction ofGoss Orientation

Grain Growth

As shown in Fig.5, the temperature at 400 °C is a turn-

ing point of the transformation from deformation textures

to recrystallization textures, which is corresponding to the

dominative component from copper {112} 〈111〉 to Goss

{110} 〈001〉. The Goss component becomes strong as the

temperature increases. Previous researches show that during

the grain growth after the primary recrystallization, there

is an exaggerated growth of some grains with an orienta-

tion advantaged at the expense of other newly formed grains

[43]. The principal thermodynamic driving force of the grain

growth is the tendency to diminish the overall grain bound-

ary ‘surface’ energy [48]. Thus, as the grains grow in size

and decrease in numbers, the grain boundary area diminishes

and the total surface energy is lowered accordingly [48]. In

addition, the certain oriented grains have a growth advan-

tage to other grains [49], and the strong recrystallization

texture develops. As shown in Fig.9a, the magniﬁcation

of local Position 4 in Fig.3f, the growth of Goss grains

has an obvious advantage than others in size. In Fig.9b,

the HAGBs were shown in black lines, the LAGBs were

shown in white lines, and the twin boundaries were shown

Fig. 7 Grain boundary map of the local position 2 in Fig.3c. The HAGBs, Σ3, and Σ9 twin boundaries are represented by black, red, and pink

lines, respectively

Fig. 8 Grain boundary map of the local position 3 in Fig. 3d. The

HAGBs, Σ3, and Σ9 twin boundaries are represented by black, red,

and pink lines, respectively

S.-W.Wang et al.

1 3

in red lines, respectively. The rapid growing of orientated-

advantage Goss grains will encounter each other and form

LAGBs (shown as the black arrows) with further increasing

annealing temperatures. This will signiﬁcantly decrease the

fraction of HAGBs and the fraction of Σ3 concurrently, as

shown in Fig.4. Figure9b illustrates the grains combining

process, and similar result was reported in Cu-45at.% Ni

alloy [50] that during grain growth the random-orientated

grains are consumed by cube-oriented grains, which leads

to the obvious decrease of HAGBs. During this combining

process, the twin of type 3 marked in red circle was left

inner the grown grain and thus transforms to type 1 twin. It

is assumed that the left twins will be absorbed by the growth

of grain as the temperature continues to rise, or they will be

pinned by the impurities and preserved in the matrix.

5 Conclusions

(1) The fraction of Σ3 boundaries increases sharply at the

early stage of heat treatment, which is consistent with

the variation trend of HAGBs. The migration of the

HAGBs and minimum grain boundary energy theory

will contribute to the formation of large number of

annealing twins during recrystallization.

(2) At the middle stage of recrystallization process,

the migration rate of grain boundaries decreases on

account of the grain growth; meanwhile, the unique

Σ9 boundaries are formed due to the interaction of the

Σ3 boundaries. As a result, the Σ3 boundaries as well

as the HAGBs decrease rapidly.

(3) During the grain coarsening stage, a strong recrystalli-

zation texture was formed due to the fact that the grains

of Goss orientation have a growth advantage over the

others. These grains encounter with each other by trans-

forming the common HAGBs into LAGBs as well as

consuming the left twins to further growth, which leads

to the decrease of the fraction of HAGBs and the frac-

tion of Σ3 concurrently.

Acknowledgements This work was financially supported by the

Natural Science Foundation of Shandong Province under Grant No.

ZR2018MEE005.

References

[1] H.C.H. Carpenter, S. Tamura, Proc. R. Soc. Lond. A 113, 28

(1926)

[2] D. Field, L. Bradford, M. Nowell, T. Lillo, Acta Mater. 55, 4233

(2007)

[3] T.H. Chuang, H.C. Wang, C.H. Tsai, C.C. Chang, C.H. Chuang,

J.D. Lee, H.H. Tsai, Scr. Mater. 67, 605 (2012)

[4] B. Lin, Y. Jin, C.M. Heﬀeran, S.F. Li, J. Lind, R.M. Suter, M.

Bernacki, N. Bozzolo, A.D. Rollett, G.S. Rohrer, Acta Mater. 99,

63 (2015)

[5] D.G. Brandon, Acta Mater. 14, 1479 (1966)

[6] V. Randle, Acta Mater. 52, 4067 (2004)

[7] V. Randle, Mater. Sci. Technol. 26, 774 (2010)

[8] T. Watanabe, J. Mater. Sci. 46, 4095 (2011)

[9] K. Lu, L. Lu, S. Suresh, Science 324, 349 (2009)

[10] C.A. Stein, A. Cerrone, T. Ozturk, S. Lee, P. Kenesei, H. Tucker,

R. Pokharel, J. Lind, C. Heﬀeran, R.M. Suter, A.R. Ingraﬀea, A.D.

Rollett, Curr. Opin. Solid St. M. 18, 244 (2014)

[11] E.A. Holm, S.M. Foiles, Science 328, 1138 (2010)

[12] W.G. Burgers, C.G. Dunn, F. Lionetti, A.J. Shaler, L.D. Jaﬀe,

Trans. AIMME 185, 860 (1949)

[13] W.G. Burgers, J.C. Meijs, T.J. Tiedema, Acta Mater. 1, 75 (1953)

[14] S. Dash, N. Brown, Acta Mater. 11, 1067 (1963)

[15] H. Gleiter, Acta Mater. 17, 1421 (1969)

[16] S. Mahajan, C.S. Pande, M.A. Imam, B.B. Rath, Acta Mater. 45,

2633 (1997)

[17] C.S. Pande, M.A. Imam, B.B. Rath, Met. Trans. A 21, 2891 (1990)

[18] R.L. Fullman, J.C. Fisher, J. Appl. Phys. 22, 1350 (1951)

[19] P.J. Goodhew, Metal Sci. 13, 108 (1979)

[20] M.A. Meyers, L.E. Murr, Acta Mater. 26, 951 (1978)

[21] L.E. Murr, J. Appl. Phys. 39, 5557 (1968)

Fig. 9 IPF map and grain boundary map of the local Position 4 in Fig.4e: a IPF, b grain boundary map. The HAGBs, LAGBs, and Σ3 twin

boundaries are represented by black, white, and red lines, respectively

Evolution ofAnnealing Twins andRecrystallization Texture inThin-Walled Copper Tube During…

1 3

[22] X.P. Chen, L.F. Li, H.F. Sun, L.X. Wang, Q. Liu, Mater. Sci. Eng.,

A 622, 108 (2015)

[23] X.M. Chen, Y.C. Lin, F. Wu, J. Alloys Compd. 724, 198 (2017)

[24] W. Wang, S. Lartigue-Korinek, F. Brisset, A.L. Helbert, J. Bour-

gon, T. Baudin, J. Mater. Sci. 50, 2167 (2014)

[25] E. Niloofar, Z.H. Abbas, S. Amirali, A.L. Helbert, B. Thierry,

Adv. Eng. Mater. 20, 1700928 (2018)

[26] S. Suwas, R.K. Ray, Crystallographic Texture of Materials, 1st

edn. (Brian Derby, Manchester, 2014)

[27] C.F. Gu, L.S. Toth, Y.D. Zhang, M. Hoﬀman, Scr. Mater. 51, 92

(2014)

[28] J.J. Sidor, L.A.I. Kestens, Scr. Mater. 273, 68 (2013)

[29] Z.N. Mao, R.C. Gu, F. Liu, Y. Liu, X.Z. Liao, J.T. Wang, Mater.

Sci. Eng., A 186, 674 (2016)

[30] G. Anand, K. Barai, R. Madhavan, P.P. Chattopadhyay, Mater. Sci.

Eng., A 114, 638 (2015)

[31] L.F. Shuai, T.L. Huang, G.L. Wu, X. Huang, O.V. Mishin, J.

Alloys Compd. 399, 749 (2018)

[32] J.H. Choi, S.Y. Kang, D.N. Lee, J. Mater. Sci. 4055, 35 (2000)

[33] T. Baudin, A.L. Etter, R. Penelle, Mater. Charact. 947, 58 (2007)

[34] N.A. Hamdany, H.G. Brokmeier, M. Salih, Z.Y. Zhong, B.

Schwebke, N. Schell, W. Gan, Mater. Charact. 125, 139 (2018)

[35] S.W. Wang, S.H. Zhang, H.W. Song, Y. Chen, Chin. J. Nonferrous

Met. 4, 782 (2019)

[36] S.W. Wang, Y. Chen, H.W. Song, A.A. El-Aty, J.S. Liu, S.H.

Zhang, Int. J. Mater. Form. (2020). https ://doi.org/10.1007/s1228

9-020-01538 -z

[37] K.H. Song, Y.B. Chun, S.K. Hwang, Mater. Sci. Eng., A 454, 629

(2007)

[38] K.K. Alaneme, E.A. Okotete, J. Sci.: Adv. Mater. Devices 4, 19

(2019)

[39] Q.Y. He, T.L. Huang, L.F. Shuai, Y.B. Zhang, G.L. Zhang, X.X.

Huang, D.J. Jensen, Scr. Mater. 153, 68 (2018)

[40] G.L. Wu, D.J. Jensen, Acta Mater. 55, 4955 (2007)

[41] T.J. Sabin, G. Winther, D.J. Jensen, Acta Mater. 51, 3999 (2003)

[42] S. Mahajan, Scr. Mater. 68, 95 (2013)

[43] F.J. Humphreys, M. Hatherly, Recrystallization and Related

Annealing Phenomena, 2nd edn. (Pergamon, New York, 2004)

[44] W.X. Chen, C. Jia, B.J. Hu, C.W. Zheng, D.Z. Li, Mater. Sci. Eng.,

A 733, 419 (2018)

[45] W.L. Grube, S.R. Rouze, Can. Metall. Quart. 2, 31 (1963)

[46] L.C. Lim, R. Raj, Acta Mater. 32, 1177 (1984)

[47] H. Beladi, P. Cizek, P.D. Hodgson, Metall. Mater. Trans. A 40,

1175 (2009)

[48] H. Kim, C. Kang, M. Huh, O. Engler, Scr. Mater. 57, 325 (2007)

[49] P.R. Rios, G. Gottstein, L.S. Shvindlerman, Mater. Sci. Eng., A

332, 231 (2002)

[50] H. Tian, H.L. Suo, O.V. Mishin, Y.B. Zhang, D.J. Jensen, J.C.

Grivel, J. Mater. Sci. 48, 4183 (2013)